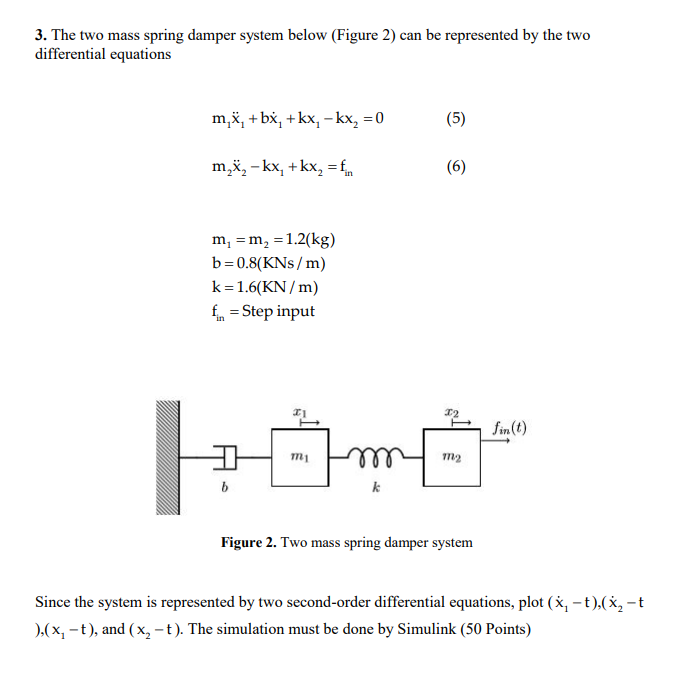
Two Mass Spring System Differential Equation

Now using Newtons law F m a and the definition of acceleration as a x we can write two second order differential equations. Where x displacement from equilibrium position m t time s m mass 20 kg c damping coefficient Nsm k spring constant Nm. Two-Mass System Derive the equation of motion for x 2 as a function of F a. The spring is in compression and the connecting-spring force magnitude is. Lets start with the model for the second mass-spring component. BACKGROUND MATH The time domain equation of motion for the mass-spring-damper is represented by Newtons Second Law written as the fol-lowing force balance on a structure 𝐌 𝐱 𝐂 𝐱 𝐊 𝐱 M Mass C Damping K Stiffness. The equations that govern a mass-spring system At equilibrium.

M1 x1 k1 x1 R1 k2 x2 x1 w1 R2 m2 x2 k2 x2 x1 w1 R2.

Say a wall far away. The indicated damping is viscous. X Ax Need to obtain expression for dv 1dt in terms of V 1 and i L Need to obtain expression for di. In layman terms Lissajous curves appear when an objects motions have two independent frequencies. Thats why we can write F_1equiv Mfracd2x_1dt2 kx_1kx_2-l Where x_1 and x_2 means the position of the first M and second m mass with respect our origin. Now we consider a mass on a spring in which there is friction.

Solved 4 The Two Mass Spring Damper System Below Can Be Chegg Com

Two mass spring system differential equation. Energy in the Ideal Mass-Spring System. The equations that govern a mass-spring system At equilibrium. M u γ u k u Ft This is a second order linear differential equation with constant coefficients.

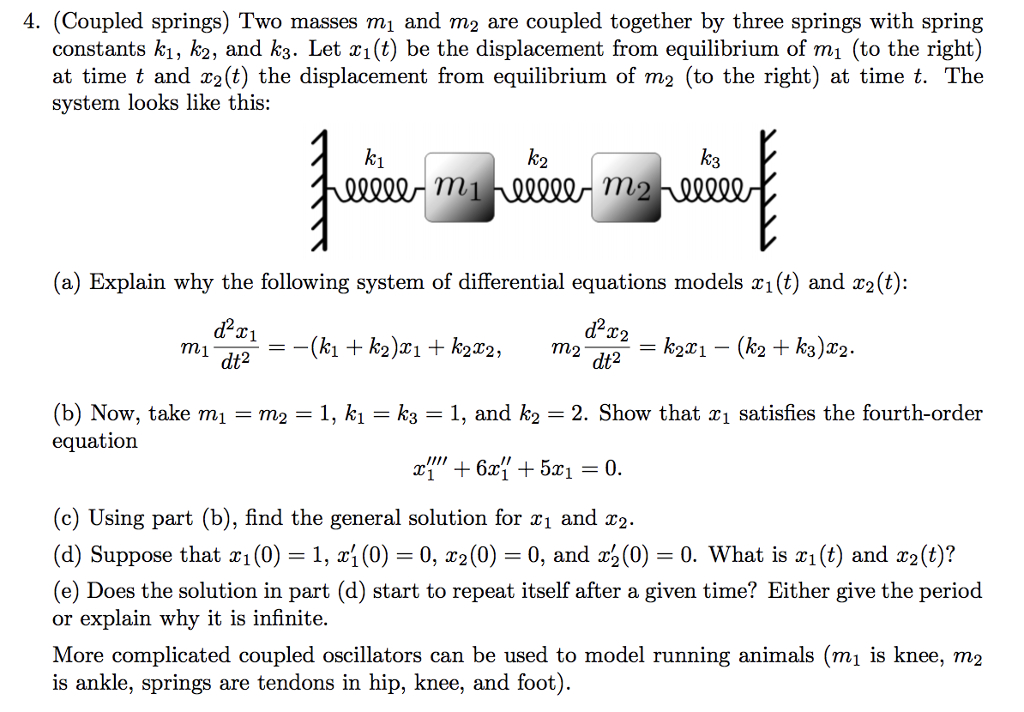
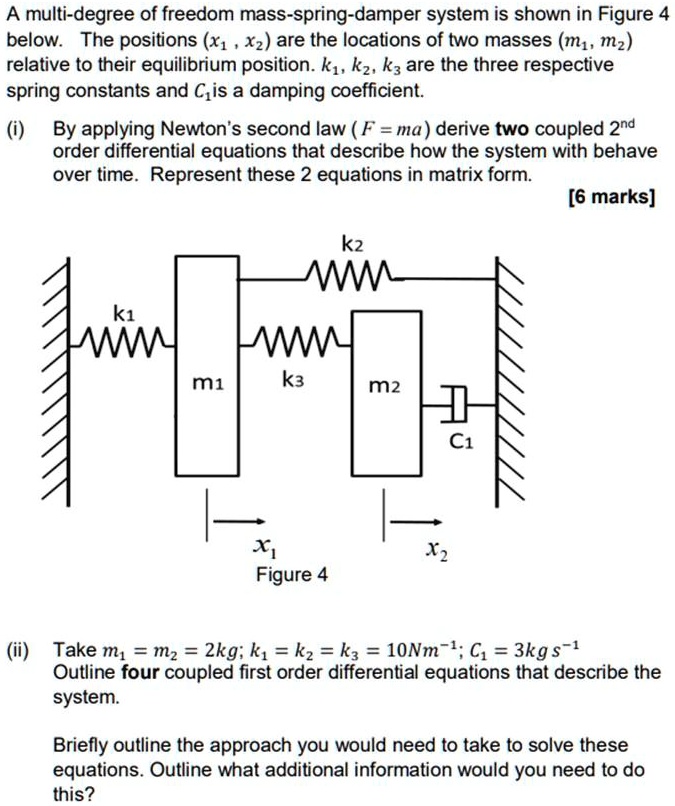
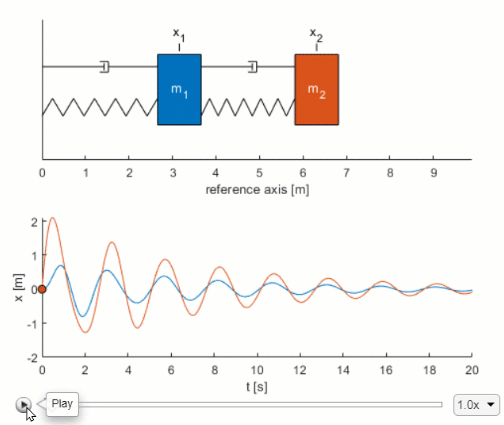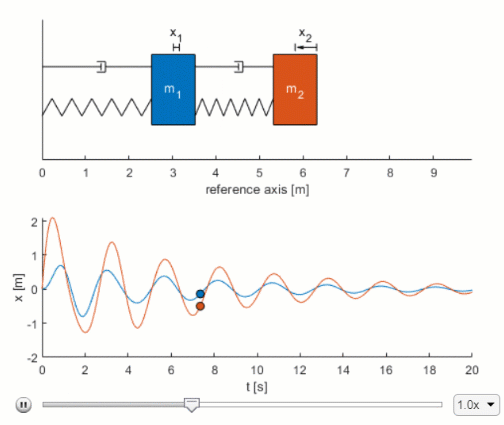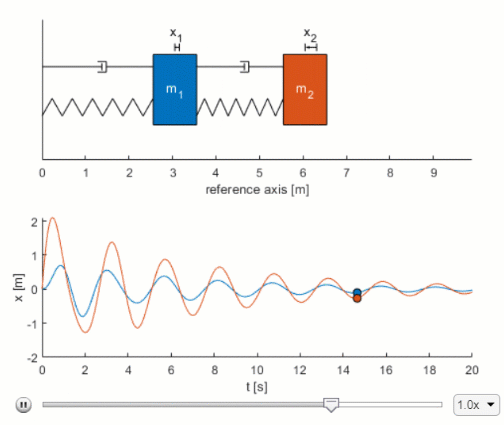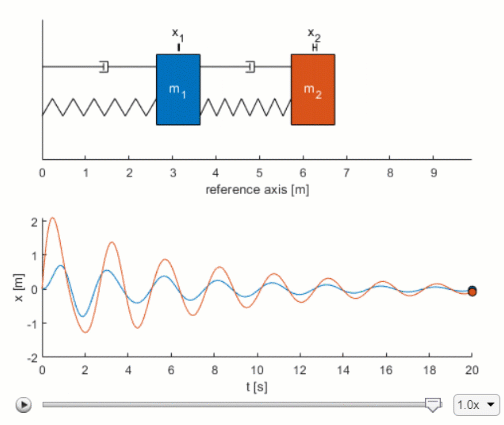
The spring force tension is positive is F k x_2-x_1 and the two equations of motion beginalign F m_1 ddotx_1 -F m_2 ddotx_2 endalign All this is trivial. The equation of motion of a damped spring-mass system is given by the 2nd order ordinary differential equation GDP as follows. BACKGROUND MATH The time domain equation of motion for the mass-spring-damper is represented by Newtons Second Law written as the fol-lowing force balance on a structure 𝐌 𝐱 𝐂 𝐱 𝐊 𝐱 M Mass C Damping K Stiffness.

To do the equations of motion you need positions from an inertial reference frame. C2 bm c km exp ct 0. Types of Solution of Mass-Spring-Damper Systems and their Interpretation The solution of mass-spring-damper differential equations comes as the sum of two parts.

By Hookes Law mg kL While in motion. This is the full blown case where we consider every last possible force that can act upon the system. Eytan Modiano Slide 3 Second order RC circuit System with 2 state variables Described by two coupled first-order differential equations States Voltage across the capacitor - V 1 Current through the inductor - i L What to obtain state equations of the form.

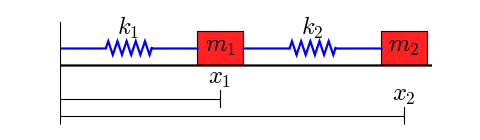
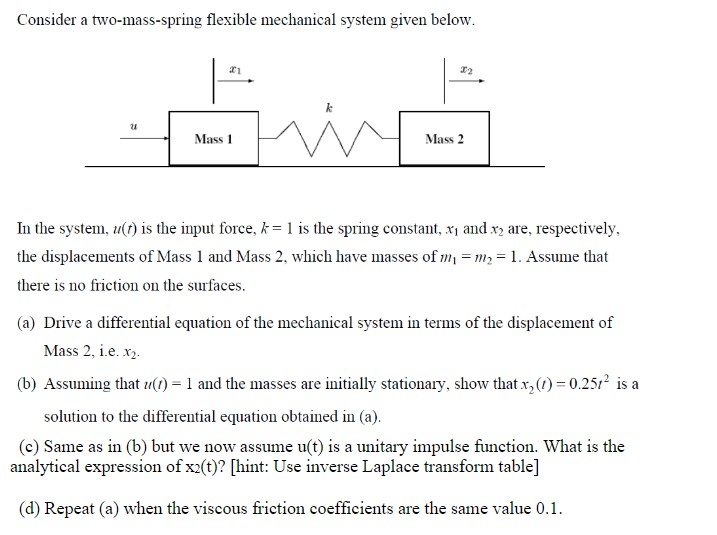
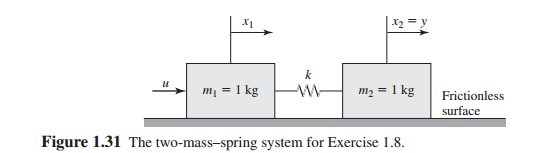
It usually comes with two initial conditions. L2 x2 x1 w1 R2. Two-Mass System Derive the equation of motion for x 2 as a function of F a.

F t l y dt dy R dt d y M λ 2 2. Equations of Motion Assuming. Lets start with the model for the second mass-spring component.

Ut 0 u 0 and ut 0 u 0. Is the characteristic or natural angular frequency of the system.

Coupled Spring Mass System Scipy Cookbook Documentation

Differential Equation Modeling Spring And Mass Sharetechnote

Solved Consider A Two Mass Spring Flexible Mechanical System Chegg Com

Differential Equation Modeling Spring And Mass Sharetechnote

Mec3403

Illustration Of Two Mass Spring System Download Scientific Diagram

How To Design Two Mass Damper Spring System In Simulink Youtube

Differential Equation Modeling Spring And Mass Sharetechnote

General 2 Dof Mass Spring Damper System Download Scientific Diagram

Mass Spring System

Transfer Function For Double Cart System Mathematics Stack Exchange

Modeling A Mass Spring Damper System Physics Forums

2dof Mass Spring Damper System Subject To Stochastic Base Excitation X Download Scientific Diagram

Double Spring Mass Systems Matlab S Ode 45

Pdf On A Differential Equation With Caputo Fabrizio Fractional Derivative Of Order 1 Lt Beta Leq 2 And Application To Mass Spring Damper System Semantic Scholar

Differential Equation Modeling Spring And Mass Sharetechnote

Get Answer Show Transcribed Image Text 2 Coupled Mass Spring System A Write Transtutors

Spring Mass System An Overview Sciencedirect Topics

Coupled Oscillators Coordinates Ptw Youtube

Tuned Mass Spring Damper Design Application Center

Compute The Damping Value For Two Masses In A Harmonic Oscillator Physics Stack Exchange

302 Vibration Of Mass Spring Damper System 2 Memorandums Of A Novice Automotive Engineer

Solved 4 Coupled Springs Two Masses M And M2 Are Coupled Chegg Com

1

Solved Let Us Consider An Aging Spring Mass System Where The Restoring Force Of The Spring And The Damping Force Are Both Weakening Exponentially Course Hero

Dynamics Of A Double Spring Mass Wolfram Demonstrations Project

Ebook Engineering Analysis Using Scilab And C

Modal Analysis

Scielo Brasil Analytic Solution To The Motion Of Mass Spring Oscillator Subjected To External Force Analytic Solution To The Motion Of Mass Spring Oscillator Subjected To External Force

Solved A Coupled Spring Mass System Is Shown In Figure P2 3 The Masses And S Solutioninn

Analytical Results Sarahandxingchen 421lab5

Differential Equation Modeling Spring And Mass Sharetechnote

Mass Spring Damper System Tutorial Test 1 Derive The Differential Equation And Find The Transfer Function For The Following Mass Spring Damper System Ppt Download

Solved Consider The Two Mass Spring System Depicted In Figure 1 31 A 1 Answer Transtutors

Ebook Dynamic System Modeling And Control

Mec3403

Dynamics Of A Double Spring Mass Wolfram Demonstrations Project

Double Spring Mass Systems Matlab S Ode 45

Differential Equations Modeling

Solved Multi Degree Of Freedom Mass Spring Damper System Is Shown In Figure Below The Positions X1 Xz Are The Locations Of Two Masses Mz Mz Relative To Their Equilibrium Position K1 Kz Kz Are The

Solved 3 The Two Mass Spring Damper System Below Figure 2 Chegg Com

Coupled Differential Equations How To Write In Terms Of Only One Coordinate Physics Stack Exchange

Diff Eqn Mass Spring Problem Example Youtube

Consider A Mass Spring Damper System I E The Plant Described By The Following Second Order Differential Equation Wh Homeworklib

Mass Spring Damper Systems File Exchange Matlab Central

Expm 1

State Space Representation Ppt Download

1 9 The Mass Damper Spring System A 2nd Order Lti System And Ode Engineering Libretexts

1

In this case the differential equation governing the motion would be simply k 0 xx m.

The spring is in compression and the connecting-spring force magnitude is. The right hand side encodes the INPUT SIGNAL an external force at work. Rearranging these differential equations gives Eqs3122. Here we have made use of the fact that a mass attached to the left end of a spring of extension and spring constant experiences a horizontal force whereas a mass attached to the right end of the same spring experiences an equal and opposite force. It usually comes with two initial conditions. Now using Newtons law F m a and the definition of acceleration as a x we can write two second order differential equations. F t l y dt dy R dt d y M λ 2 2. The complementary function which arises solely due to the system itself and the particular integral which arises solely due to the applied forcing term. Ill hint at two of the three.

Lets start with the model for the second mass-spring component. F t l y dt dy R dt d y M λ 2 2. So the di erential equation for the mass spring is now m d2x dt2 kx dx zdt friction force. In layman terms Lissajous curves appear when an objects motions have two independent frequencies. Types of Solution of Mass-Spring-Damper Systems and their Interpretation The solution of mass-spring-damper differential equations comes as the sum of two parts. The force is considered to be proportional the instantaneous velocity. BACKGROUND MATH The time domain equation of motion for the mass-spring-damper is represented by Newtons Second Law written as the fol-lowing force balance on a structure 𝐌 𝐱 𝐂 𝐱 𝐊 𝐱 M Mass C Damping K Stiffness.